极值点偏移
简介 #
已知 $f(x_1)=f(x_2)$,$x_0$ 是极值点,求证 $x_1+x_2>2x_0$ 或 $x_1x_2>x_0^2$. 这就是极值点偏移问题.
$$极值点不偏移$$

$$\frac{x_1+x_2}{2}=x_0$$
$$极值点左偏$$
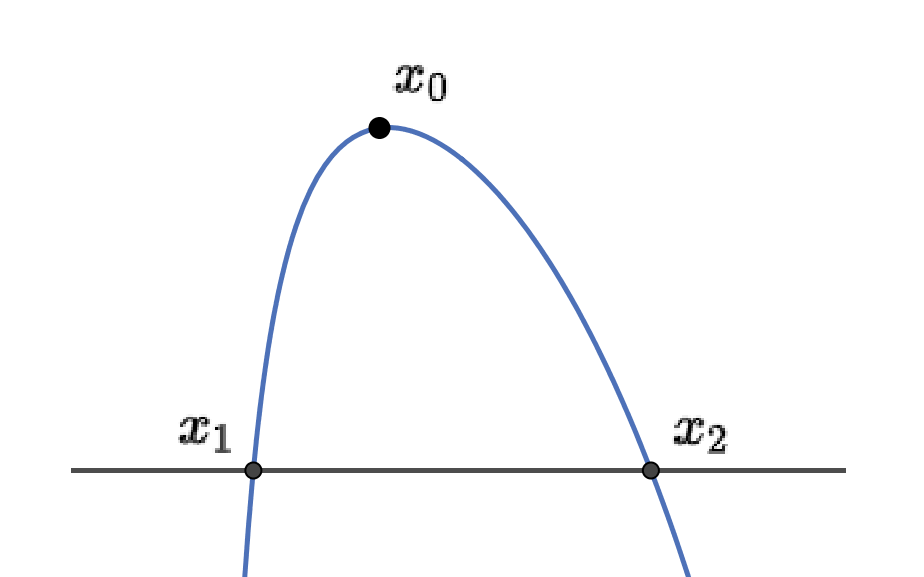
$$\frac{x_1+x_2}{2}>x_0$$
$$极值点右偏$$
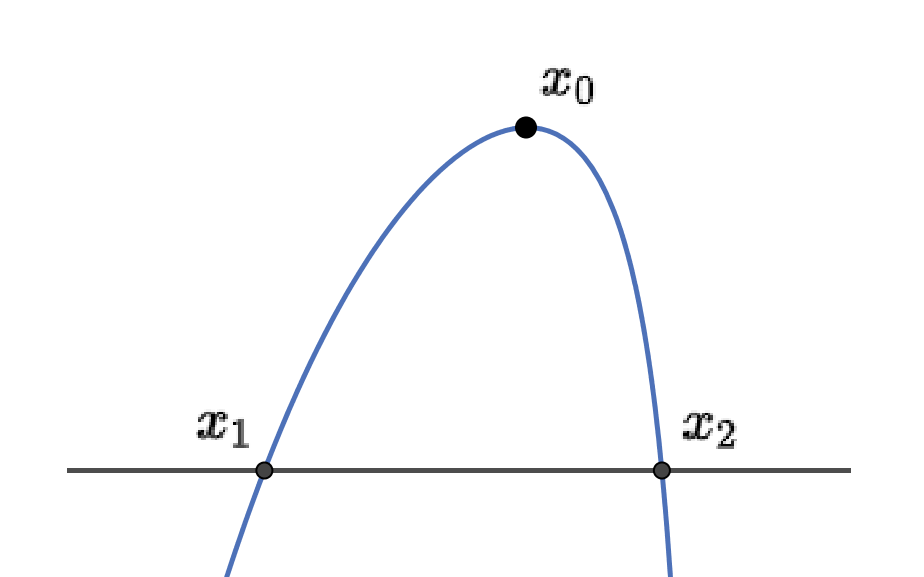
$$\frac{x_1+x_2}{2}<x_0$$
原理 #
以极值点左偏为例,求证 $x_1+x_2>2x_0$,即 $x_1>2x_0-x_2$.
若 $x_1$ 和 $m-x_2$ 在函数的 $\uparrow$ 区间,则 $f(x_2)>f(2x_0-x_2)$.
原命题转化为关于 $x_2$ 的单变量问题.
$x_1x_2>x_0^2$ 同理.
例题 #
$f(x)=\frac{x}{e^x}$,若 $f(x_1)=f(x_2)$ 且 $x_1\not=x_2$,求证 $x_1+x_2>2$.
证
$f’(x)=\frac{1-x}{e^x}$.
- $x\in(-\infty,1),f’(x)>0,f(x)\uparrow$.
- $x\in(1,+\infty),f’(x)<0,f(x)\downarrow$.
不妨设 $x_1<1<x_2$.
$$x_1+x_2>2 \eq x_1>2-x_2 \eq f(x_1)>f(2-x_2) \eq f(x_2)>f(2-x_2)$$
于是转而证明 $f(x_2)-f(2-x_2)>0$,其中 $x_2>1$.
设 $g(x)=f(x)-f(2-x)=\frac{x}{e^x}-\frac{2-x}{e^{2-x}}$,即证 $g(x)$ 在 $(1,+\infty)$ 上恒 $>0$.
$g’(x)=\frac{1-x}{e^x}-\frac{1-x}{e^{2-x}}=(x-1)(e^{x-2}-e^{-x})$.
$\therefore x\in(1,+\infty),g’(x)>0,g(x)\uparrow$.
$\therefore g(x)>g(1)=f(1)-f(1)=0$. 命题得证.